Chapter 2 - Kinematics: Motion in One Dimension

Sections 2.1 - 2.5 : Velocity and Instantaneous Velocity

Here we define velocity, explain how velocity differs from speed and learn the average velocity calculation. From there, we show graphically what instantaneous velocity is, how the slope of the curve of distance vs. time is used to determine it, and how it is approached as we look at smaller and smaller intervals of time. Then we see how to derive the instantaneous velocity from an equation, by using incremental time and distance, and ultimately end up with the same result as had we simply differentiated the equation.

Section 2.6 : Acceleration

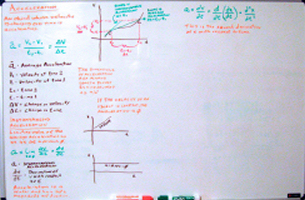
Here we define acceleration, and learn the average acceleration calculation. From there, we show graphically what instantaneous acceleration is, how the slope of the curve of velocity vs. time is used to determine it, and how it is approached as we look at smaller and smaller intervals of time.

Section 2.7 : Uniformly Accelerated Motion

Show how to relate average acceleration to instantaneous acceleration and average velocity to instantaneous velocity. Then use these realtionships and some additional logic to derive 4 very useful equations tying position, velocity, acceleration and time together.







This is a practical example of the use of some of the acceleration equations. We solve a problem that deals with the size a crumple zone must be on the front of a car for a passanger to survive a crash.








This is a fun use for these equations. In Robert Heinlein's "Have Space Suit Will Travel", the main character is assessing in his mind how to steal a ship and get home... from Pluto! He has been taken there at 8g s of acceleration. It took something like 5 days. He is wondering how long it would take him to return to Earth if he made the trip at 1g. In this calculation we figure the velocity attained at the half way point for an 8g trip and a 1g trip, and show the time that each would take. Considering that the calculation here assumed Pluto was close to 20% further out than its perihelion distance, the calculated 8g time is right in line with the book's 5 days for the full trip!

Section 2.8 : Motion of Falling Bodies - Parts 1 and 2

Using an example that asks five questions, we see how to determine position, time and velocity in a falling bodies problem. This illustration covers questions one and two of five.

Section 2.8 : Motion of Falling Bodies - Parts 3 through 5

Continuing with the example that asks five questions, we see how to determine position, time and velocity in a falling bodies problem. This illustration covers questions three, four and five of five.







Another example of a falling body problem that makes use of the acceleration equations for a solution. This one has an object released from an ascending aircraft as its subject.









Here we take a real world example of a slingshot to illustrate the uses for several of the concepts and equations discussed so far. It is interesting to see true quantites defined for acceleration, time and velocity for the simple act of shooting a marble into the air and watching it fall back to earth.

Section 2.9 : Variable Acceleration and Graphical Analysis, with the use of Calculus

Here we related graphical representations of variable acceleration and the equations that can be derived from them. Integral calculus is introduced to show how to sum up an infinite number of infinitesimally small areas to arrive at a displacement.







And here we demonstrated an example of the use of the integration technique by figuring out the velocity and displacement of an object based on a given equation involving variable acceleration.